Two Samples Estimation: Diff Btw Two Means

“확률과 통계(MATH230)” 수업에서 배운 것과 공부한 것을 정리한 포스트입니다. 전체 포스트는 Probability and Statistics에서 확인하실 수 있습니다 🎲

Interval Estimation 포스트에서 다룬 <Interval Estimation>을 특정 상황에 어떻게 적용할 수 있는지를 다루는 포스트입니다.

Two Samples Estimation

Supp. there are two populations and assume that both follow some random distributions with means $\mu_1$ and $\mu_2$ and variances $\sigma_1^2$, $\sigma_2^2$ respectively.

Take random samples $X_1, \dots, X_{n_1}$ and $Y_1, \dots, Y_{n_2}$, and assume that $X_i$s and $Y_j$s are independent.

Supp. that their observed sample means are $\bar{x}$ and $\bar{y}$, and their sample variances are $s_1^2$ and $s_2^2$.

✨ Goal: Find $100(1-\alpha)\%$ confidence interval for $\mu_1 - \mu_2$.

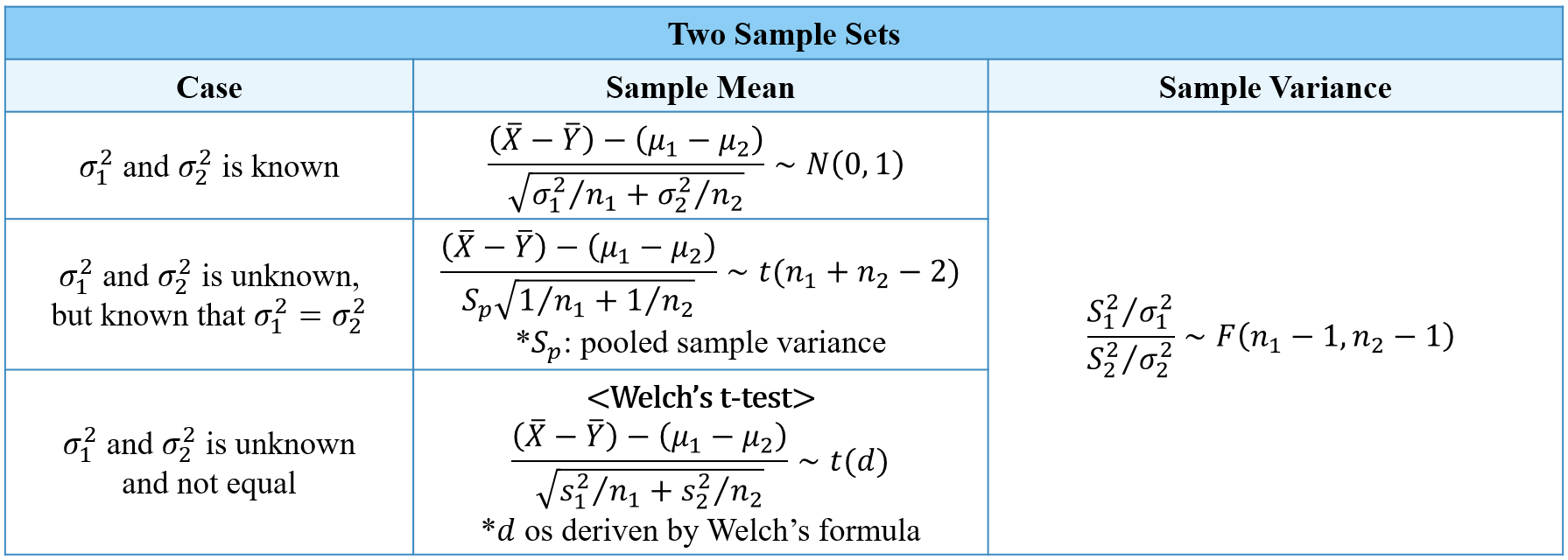
$\sigma_1^2$ and $\sigma_2^2$ are known

By CLT, $\bar{X} \overset{D}{\approx} N(\mu_1, \sigma_1^2 / n_1)$ and $\bar{Y} \overset{D}{\approx} N(\mu_2, \sigma_2^2 / n_2)$, in addition $\bar{X} \perp \bar{Y}$.

Then,

\[\bar{X} - \bar{Y} \overset{D}{\approx} N(\mu_1 - \mu_2, \; \sigma_1^2 / n_1 + \sigma_2^2 / n_2)\]

then, the confidence interval is

\[\left( \bar{x} - \bar{y} - z_{\alpha/2} \cdot \sqrt{\frac{\sigma_1^2}{n_1} + \frac{\sigma_2^2}{n_2}} , \; \bar{x} - \bar{y} + z_{\alpha/2} \cdot \sqrt{\frac{\sigma_1^2}{n_1} + \frac{\sigma_2^2}{n_2}} \right)\]

💥 이때, 주의할 점은 이것은 true interval이 아니라 approximate interval이라는 점이다; by CLT

💥 또, 이 근사는 $X_i$, $Y_j$가 모두 iid normal이여야 가능하다!

$\sigma_1^2$ and $\sigma_2^2$ are unknown, but known that $\sigma_1^2 = \sigma_2^2$

앞에서 우리는 CLT를 사용해 $\frac{\bar{X} - \mu}{\sigma / \sqrt{n}}$를 사용했다. 하지만, 이번에는 정확한 $\sigma^2$의 값을 알지 못한다. 그래서 $\sigma^2$ 대신 sample variance $s^2$을 사용한다!!

이때, 두 표본이 $\sigma^2$를 공유하므로 둘을 종합한 값으로 sample variance를 잡아야 한다. 이것이 바로 “Pooled Sample Variance $S_p^2$”다.

Definition. pooled sample variance

\[\begin{aligned} S_p^2 &= \frac{\displaystyle \sum^{n_1}_1 (X_i - \bar{X})^2 + \sum^{n_2}_1 (Y_i - \bar{Y})^2}{n_1 - 1 + n_2 - 1} \end{aligned}\]

위의 식을 잘 변형해보면 아래와 같다.

\[S_p^2 = \frac{(n_1 - 1) \cdot S_1^2 + (n_2 - 1) \cdot S_2^2}{(n_1 - 1 + n_2 - 1)}\]

샘플의 분산은 카이제곱 분포를 따르는 것을 기억하는가?

Recall. sample variance and chi-square

\[\frac{(n -1) \cdot S^2}{\sigma^2} = \sum^n \left(\frac{X_i - \bar{X}}{\sigma}\right)^2 \; \sim \; \chi^2 (n-1)\]

마찬가지로 Pooled Sample Variance로 카이제곱 분포를 따른다.

\[\frac{(n_1 - 1 + n_2 - 1) \cdot S_p^2}{\sigma^2} \overset{D}{\sim} \; \chi^2(n_1 + n_2 - 2)\]

이것은 갑자기 튀어나온게 아니고, 위의 분포가 카이제곱 분포의 선형 결합이기 때문이다.

\[\frac{(n_1 - 1) \cdot S_1^2}{\sigma^2} + \frac{(n_2 - 1) \cdot S_2^2}{\sigma^2} \; \overset{D}{\sim} \; \chi^2(n_1 - 1) + \chi^2(n_2 - 1)\]

마지막으로 추정을 위해 CLT에 대한 식을 세워야 하는데, $S_p^2$를 바탕으로 식으로 다시 쓰면,

\[\frac{(\bar{X} - \bar{Y}) - (\mu_1 - \mu_2)}{S_p \sqrt{\frac{1}{n_1} + \frac{1}{n_2}}} \; \overset{D}{\sim} t (n_1 + n_2 - 2)\]

그 다음은 지금까지 해온 것처럼 t-분포로 구간 추정을 진행하고, CI를 얻으면 된다!

* 참고로 2024-1학기 수학과 졸업시험에 이 Pooled Sample Variance에 대한 부분이 문제라고 나왔습니다;; [link]

$\sigma_1^2$ and $\sigma_2^2$ are unknown and not equal

먼저 population parameter를 기준으로 식을 세워보자.

\[\frac{(\bar{X} - \bar{Y}) - (\mu_1 - \mu_2)}{\sqrt{\frac{\sigma_1^2}{n_1} + \frac{\sigma_2^2}{n_2}}} \; \sim \; N(0, 1)\]

이때, 우리가 $\sigma_1^2$, $\sigma_2^2$를 알지 못하기 때문에 sample variance로 해당 부분을 대체한다.

\[\frac{(\bar{X} - \bar{Y}) - (\mu_1 - \mu_2)}{\sqrt{\frac{s_1^2}{n_1} + \frac{s_2^2}{n_2}}} \; \overset{D}{\sim} \; ??\]

이때, 우변이 ??인 이유는 아직까지 위의 경우에 대해 정확한 분포를 모르기 때문이다!! 그래서 이것이 어떤 DOF $d$의 t-distribution을 만족한다고 근사하여 estimation을 진행한다!

\[\frac{(\bar{X} - \bar{Y}) - (\mu_1 - \mu_2)}{\sqrt{\frac{s_1^2}{n_1} + \frac{s_2^2}{n_2}}} \; \overset{D}{\sim} \; t(d)\]

where

\[d = \frac{(s_1^2/n_1 + s_2^2/n_2)^2}{(s_1^2/n_1)^2 / (n_1-1) + (s_2^2/n_2)^2 / (n_2-1)}\]

이 근사법을 <Welch’s t-test>라고 하며, 이때 dof를 구하기 위해 사용한 식을 <Welch-Satterthwaite equation>이라고 한다.

이 경우는 t-분포의 dof를 구하기 위해 공식을 써야 하기 때문에, 왠만하면 시험에 안 나올 것이다 ㅋㅋ 오히려 나온다면, Pooled Sample Variance에 대한 부분이 나올 가능성이 크다.

맺음말

이어지는 포스트에서는 또다른 Two Samples Estimation인 <Paired Observation>의 경우를 살펴본다! 😁

👉 Two Samples Estimation: Paired Observations

지금까지의 관심사는 population mean $\mu$에 대한 추정이었다. population mean $\mu$를 추정하거나 두 population mean $\mu$의 차이값을 추정했다. 그 다음 포스트에서는 population variance $\sigma^2$를 추정한다. $\sigma^2$를 추정하거나, 두 샘플의 population variance의 비율 $\sigma_1^2 / \sigma_2^2$를 추정한다.

👉 Variance Estimation